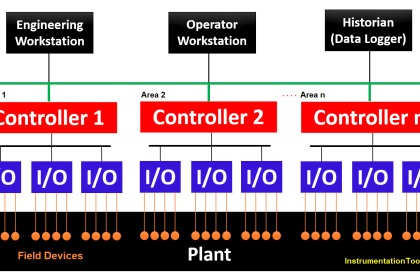
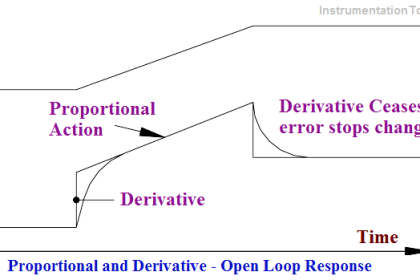
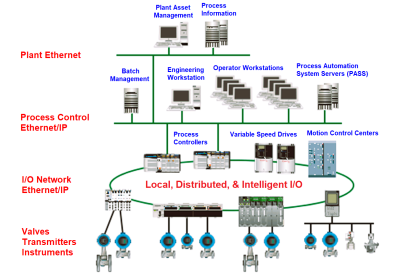
An interesting case of over-tuning is when the process variable “porpoises” (Note 1 ) on its way to setpoint following a step-change in setpoint.
Note 1 : The term “porpoise” comes from the movements of a porpoise swimming rapidly toward the water’s surface as it chases along the bow of a moving ship. In order to generate speed, the animal undulates its body up and down to powerfully drive forward with its horizontal tail, tracing a sinusoidal path on its way up to breaching the surface of the water.
The following trend shows such a response:
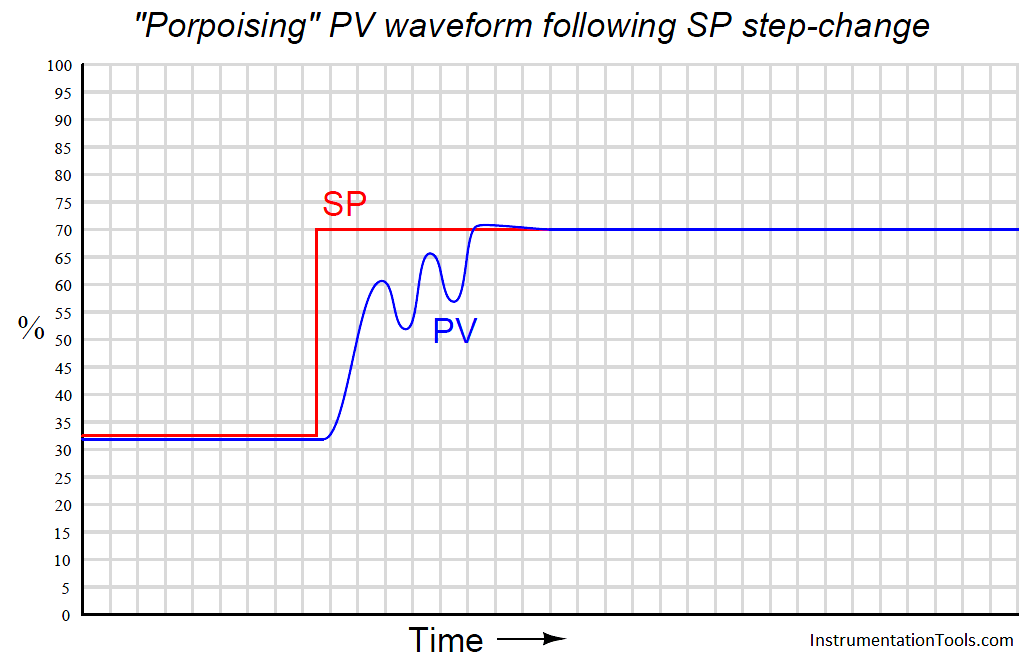
“Porpoising” is universally poor behavior for a loop, because it combines the negative consequences of over-tuning (instability and excessive valve travel) with the negative consequence of under-tuning (delay achieving setpoint). There is no practical purpose served by a loop “porpoising,” and so this behavior should be avoided if at all possible.
Thankfully, identifying the cause of “porpoising” is rather easy to do. Only two control actions are capable of causing this response: proportional and derivative. Integral action simply cannot cause porpoising. In order for the process variable to “porpoise,” the controller’s output signal must reverse direction before the process variable ever reaches setpoint. Integral action, however, will always drive the output in a consistent direction when the process variable is on one side of setpoint. Only proportional and derivative actions are capable of producing a directional change in the output signal prior to reaching setpoint.
Solely examining the process variable waveform will not reveal whether it is proportional action, derivative action, or both responsible for the “porpoising” behavior. A trial reduction in the derivative (Note 2 ) tuning parameter is one way to identify the culprit, as is phase-shift analysis between the PV and output waveforms during the “porpoising” period.
Note 2 : You could try reducing the controller’s gain as a first step, but if the controller implements the Ideal or Series algorithm, reduction in gain will also reduce derivative action, which may mask an over-tuned derivative problem.