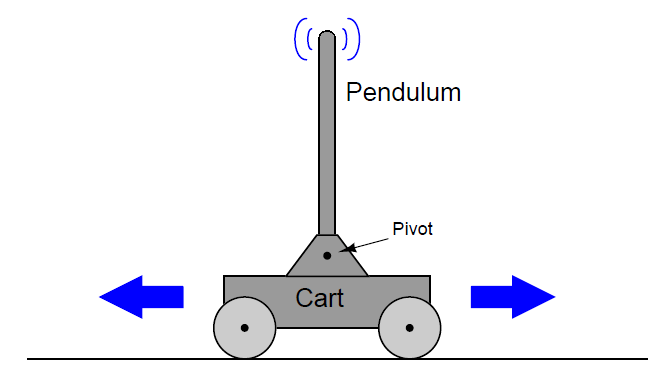
A classic “textbook” example of a runaway process is an inverted pendulum: a vertical stick balanced on its end by moving the bottom side-to-side. Inverted pendula are typically constructed in a laboratory environment by fixing a stick to a cart by a pivot, then equipping the cart with wheels and a reversible motor to give it lateral control ability. A sensor (usually a potentiometer) detects the stick’s angle from vertical, reporting that angle to the controller as the process variable. The cart’s motor is the final control element:
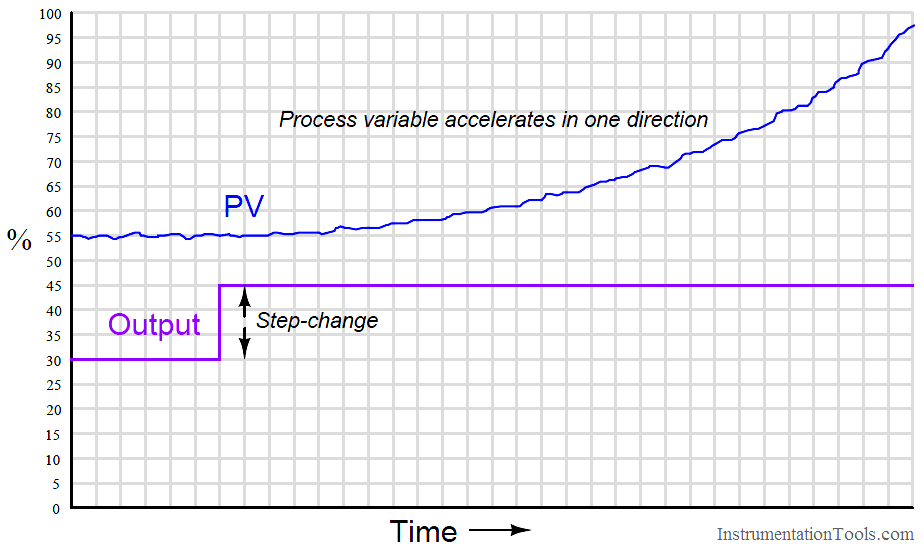
The defining characteristic of a runaway process is its tendency to accelerate away from a condition of stability with no corrective action applied. Viewed on a process trend, a runaway process tends to respond as follows to an open-loop step-change:
A synonym for “runaway” is negative self-regulation or negative lag, because the process variable curve over time for a runaway process resembles the mathematical inverse of a self-regulating curve with a lag time: it races away from the horizontal, while a self-regulating process variable draws closer and closer to the horizontal over time.
There are many examples of runaway processes in motion-control applications, especially automated controls for vertical-flight vehicles such as helicopters and vectored-thrust aircraft such.
Some chemical reaction processes are runaway as well, especially exothermic (heat-releasing) reactions. Most chemical reactions increase in rate as temperature rises, and so exothermic reactions tend to accelerate with time (either becoming hotter or becoming colder) unless checked by some external influence. This poses a significant challenge to process control, as many exothermic reactions used to manufacture products must be temperature-controlled to ensure efficient production of the desired product. Off-temperature chemical reactions may not “favor” production of the desired products, producing unwanted byproducts and/or failing to efficiently consume the reactants. Furthermore, safety concerns usually surround exothermic chemical reactions, as no one wants their process to melt down or explode.
What makes a runaway process behave as it does is internal positive feedback. In the case of the inverted pendulum, gravity pulls works to pull an off-center pendulum even farther off center, accelerating it until it falls down completely. In the case of exothermic chemical reactions, the direct relationship between temperature and reaction rate forms a positive feedback loop: the hotter the reaction, the faster it proceeds, releasing even more heat, making it even hotter. It should be noted that endothermic chemical reactions (absorbing heat rather than releasing heat) tend to be self regulating for the exact same reason exothermic reactions tend to be runaway: reaction rate usually has a positive correlation with reaction temperature.
It is easy to demonstrate for yourself how challenging a runaway process can be to control. Simply try to balance a long stick vertically in the palm of your hand. You will find that the only way to maintain stability is to react swiftly to any changes in the stick’s angle – essentially applying a healthy dose of derivative control action to counteract any motion from vertical.
Fortunately, runaway processes are less common in the process industries. I say “fortunately” because these processes are notoriously difficult to control and usually pose more danger than inherently self-regulating processes. Many runaway processes are also nonlinear, making their behavior less intuitive to human operators.
Just as integrating processes may be forced to self-regulate by the addition of (natural) negative feedback, intrinsically runaway processes may also be forced to self-regulate given the presence of sufficient natural negative feedback. An interesting example of this is a pressurized water nuclear fission reactor.
Nuclear fission is a process by which the nuclei of specific types of atoms (most notably uranium- 235 and plutonium-239) undergo spontaneous disintegration upon the absorption of an extra neutron, with the release of significant thermal energy and additional neutrons. A quantity of fissile material such as 235U or 239Pu is subjected to a source of neutron particle radiation, which initiates the fission process, releasing massive quantities of heat which may then be used to boil water into steam and drive steam turbine engines to generate electricity. The “chain reaction” of neutrons splitting fissile atoms, which then eject more neutrons to split more fissile atoms, is inherently exponential in nature. The more atoms split, the more neutrons are released, which then proceed to split even more atoms. The rate at which neutron activity within a fission reactor grows or decays over time is determined by the multiplication factor , and this factor is easily controlled by the insertion of neutron-absorbing control rods into the reactor core.
Thus, a fission chain-reaction naturally behaves as an inverted pendulum. If the multiplication factor is greater than 1, the reaction grows exponentially. If the multiplication factor is less than 1, the reaction dies exponentially. In the case of a nuclear weapon, the desired multiplication factor is as large as physically possible to ensure explosive reaction growth. In the case of an operating nuclear power plant, the desired multiplication factor is exactly 1 to ensure stable power generation.
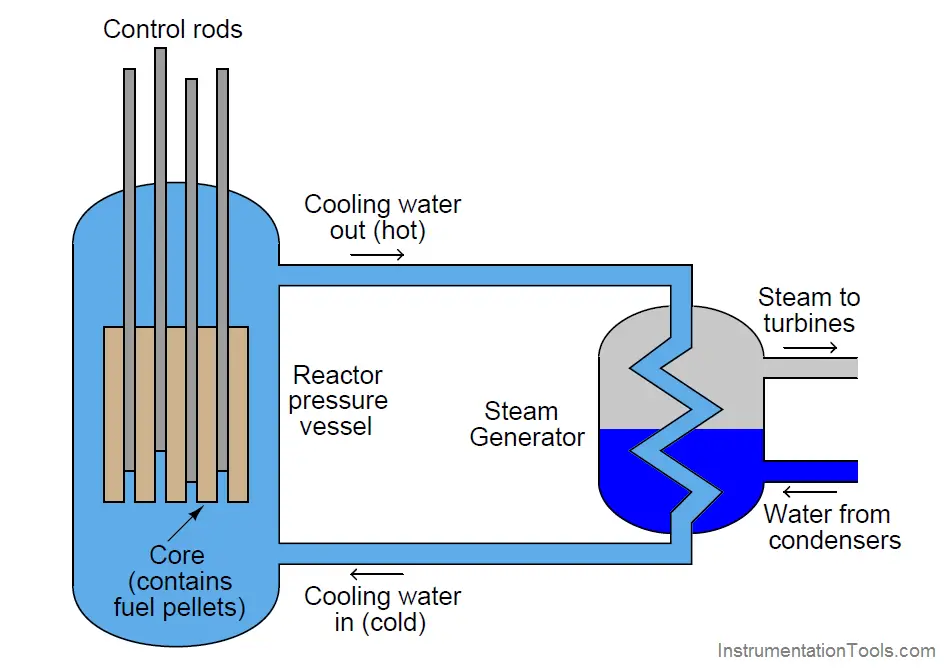
A simplified diagram of a pressurized-water reactor (PWR) is shown here:
Water under high pressure (too high of pressure to boil) circulates through the reactor vessel, carrying heat away from the nuclear core, then transferring the heat energy to a heat exchanger (“steam generator”) where a second water loop is allowed to boil into steam and drive turbine engines to spin electrical generators. Control rods inserted into the core by linear actuators adjust the multiplication factor of the reactor.
If the multiplication factor of a fission reactor were solely controlled by the positions of these control rods, it would be a classic “runaway” process, with the reactor’s power level tending to increase toward infinity or decrease toward zero if the rods were at any position other than one yielding a multiplication factor of precisely unity (1). This would make nuclear reactors extremely difficult (if not impossible) to safely control. Fortunately, there are ways to engineer negative feedback directly into the design of the reactor core so that neutron activity naturally self-stabilizes without active control rod action. In water-cooled reactors, the water itself achieves this goal. Pressurized water plays a dual role in a fission reactor: it not only transfers heat out of the reactor core and into a boiler to produce steam, but it also offsets the multiplication factor inversely proportional to temperature. As the reactor core heats up, the water’s density changes, affecting the probability of neutrons being captured by fissile nuclei. This is called a negative temperature coefficient for the reactor, and it forces the otherwise runaway process of nuclear fission to become self-regulating.
With this self-regulating characteristic in effect, control rod position essentially determines the reactor’s steady-state temperature. The further the control rods are withdrawn from the core, the hotter the core will run. The cooling water’s natural negative temperature coefficient prevents the fission reaction from “running away” either to destruction or to shutdown.
Some nuclear fission reactor designs are capable of “runaway” behavior, though. The ill-fated reactor at Chernobyl (Ukraine, Russia) was of a design where its power output could “run away” under certain operating conditions, and that is exactly what happened on April 26, 1986. The Chernobyl reactor used solid graphite blocks as the main neutron-moderating substance, and as such its cooling water did not provide enough natural negative feedback to overcome the intrinsically runaway characteristic of nuclear fission. This was especially true at low power levels where the reactor was being tested on the day of the accident. A combination of poor management decisions, unusual operating conditions, and unstable design characteristics led to the reactor’s destruction with massive amounts of radiation released into the surrounding environment. It stands at the time of this writing as the world’s worst nuclear accident.
Summary:
- Runaway processes are characterized by an exponential ramping of the process variable in response to a step-change in the control element value or load(s).
- This “runaway” occurs as a result of some form of positive feedback happening inside the process.
- Runaway processes cannot be controlled with proportional or integral controller action alone, and always requires derivative action for stability.
- Some integral controller action will be required in runaway processes to compensate for load changes.
- A runaway process will become self-regulating if sufficient negative feedback is naturally introduced, as is the case with water-moderated fission reactors.