PID Controller Loop Tuning Questions and Answers – Part 2

PID Controller Loop Tuning Questions and Answers 

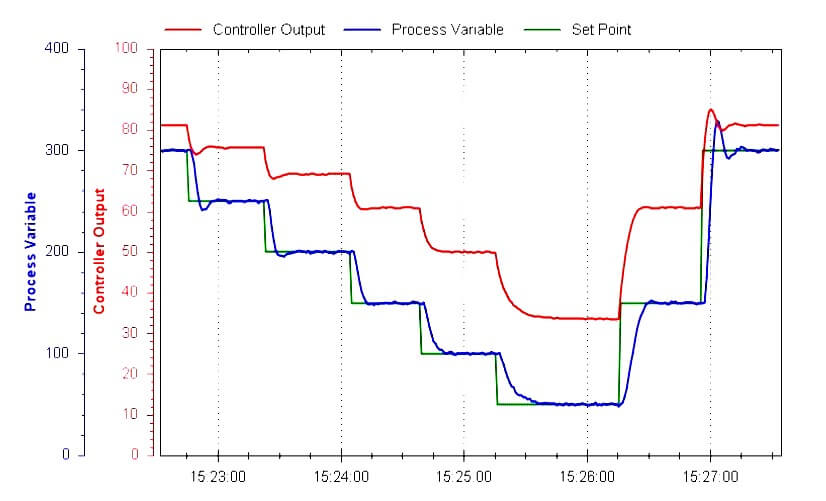
1. Examine this process trend showing the PV, SP, and Output of a loop controller:

Based on what you see here, determine the following:

• Whether this is an open-loop or a closed-loop response
• Whether the controller is (or needs to be) direct-acting or reverse-acting
• If possible, identify any problems with the field instrumentation
• If possible, identify any problems with the controller PID tuning
• Qualitatively identify the kind of PID tuning we will need for robust control

Answer :

This is a closed-loop (automatic-mode) response based on the smoothly-curved shape of the output trend in response to the process variable changing over time.

This controller is reverse-acting, as evidenced by the output trend’s inverted shape in comparison to the process variable’s trend.

No problems are evident in the field instrumentation, although it appears this process is subjected to large load changes. Note how far the output of the controller must move in order to hold the process variable relatively close to setpoint.

With all the severe load changes happening in this trend, it’s difficult to tell whether any instability is being caused by the controller (i.e. overtuning) or if the load is simply that unstable.

Several small oscillations do appear between the large surges, which may indicate an overly-aggressive controller response. Based on the phase shift between process variable and output trends being very nearly 180o , it would appear that the dominant action in this reverse-acting controller is proportional.

Perhaps the gain needs to be diminished. However, decreasing the gain too much will compromise this controller’s ability to quickly respond to severe load changes, which this process seems to suffer from.

A recommended tuning strategy would be to try some derivative action to manage load changes, while decreasing proportional action a bit. An even more important recommendation is to identify the source of the load swings, and look for ways to either minimize those variations or incorporate a different control strategy (e.g. cascade or feedforward) to better manage the variations.

2. Examine this process trend showing the PV, SP, and Output of a loop controller:

Based on what you see here, determine the following:

• Whether this is an open-loop or a closed-loop response
• Whether the controller is (or needs to be) direct-acting or reverse-acting
• If possible, identify any problems with the field instrumentation
• If possible, identify any problems with the controller PID tuning
• Qualitatively identify the kind of PID tuning we will need for robust control

Answer :

This is a closed-loop test, based on the fact the output signal responds dynamically to the changing process variable, as well as to the step-change in setpoint.

This is a reverse-acting controller: the output steps up when the setpoint steps up (implying the output would step down if the process variable stepped up).

There do not appear to be any field instrumentation problems revealed in this trend. A manual-mode (open-loop) test would be more informative in that regard, but it appears as though the process is very quick to respond with little dead time or other lags.

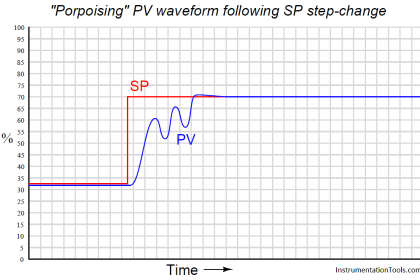
The controller tuning is clearly too aggressive for this process. Note the “porpoising” action of the PV as it approaches SP following the SP step-change. Only two types of controller action can cause this to occur: proportional, or derivative.

Porpoising is when an oscillation occurs in the PV prior to it crossing setpoint, which explains why integral action cannot ever be to blame for porpoising: the only way a loop oscillation can occur is when the final control element oscillates as well (i.e. changes direction), and since integral action will never change direction until PV crosses SP, oscillations that occur on one side of SP cannot be caused by integral action.

Looking at the phase shift between PV and output during the oscillations, it appears the output peaks may slightly lead the PV peaks, but only slightly. This suggests that proportional is the action that is too aggressive (if it were derivative, there would be more of a leading phase shift).

This is definitely a self-regulating process, as revealed by the fact a new output value is required to achieve a new setpoint value. This means integral control action will definitely be necessary. Good control will require less gain and perhaps a bit more derivative action to help cancel the lag. Integral action looks just fine where it is right now, with just a little SP overshoot.

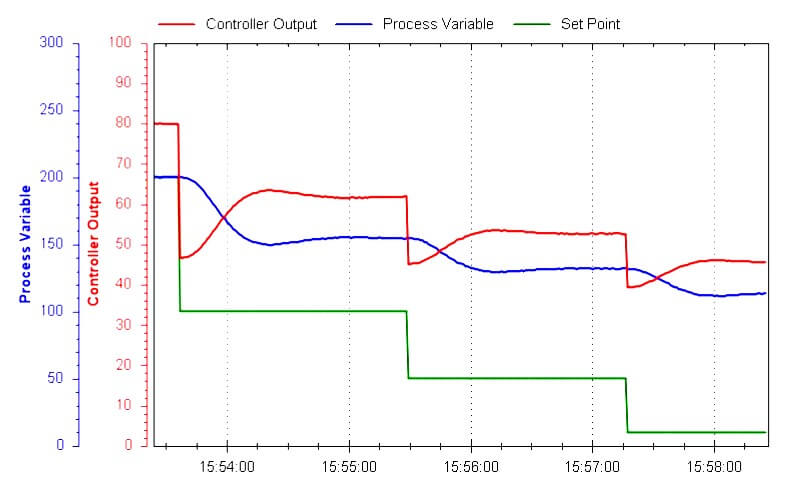
3. Examine this process trend showing the PV, SP, and Output of a loop controller:

Based on what you see here, determine the following:

• Whether this is an open-loop or a closed-loop response
• Whether the controller is (or needs to be) direct-acting or reverse-acting
• If possible, identify any problems with the field instrumentation
• If possible, identify any problems with the controller PID tuning
• Qualitatively identify the kind of PID tuning we will need for robust control

Answer :

This is a closed-loop test, based on the fact the output signal responds dynamically to the changing process variable, as well as to the step-change in setpoint.

This is a reverse-acting controller: the output steps up when the setpoint steps up (implying the output would step down if the process variable stepped up).

The only problem here is that the process exhibits a varying gain. This is why the control is oversensitive (oscillatory) at high setpoint values and sluggish at low setpoint values. This could be a function of process dynamics, or of a control valve with the wrong characteristic (e.g. an equal-percentage valve in an application better suited for a linear valve).

The controller tuning looks really good when the process variable is maintaining around 40%. This mid-range is where the tuning seems to be optimized.

This process appears to be self-regulating with short dead and lag times. It is clear from examining the phase shifts of output versus PV that there is some derivative action at work here, since the output actually leads the PV when there is oscillation. The key will be linearizing the process gain so that one set of tuning parameters will work robustly across the control range

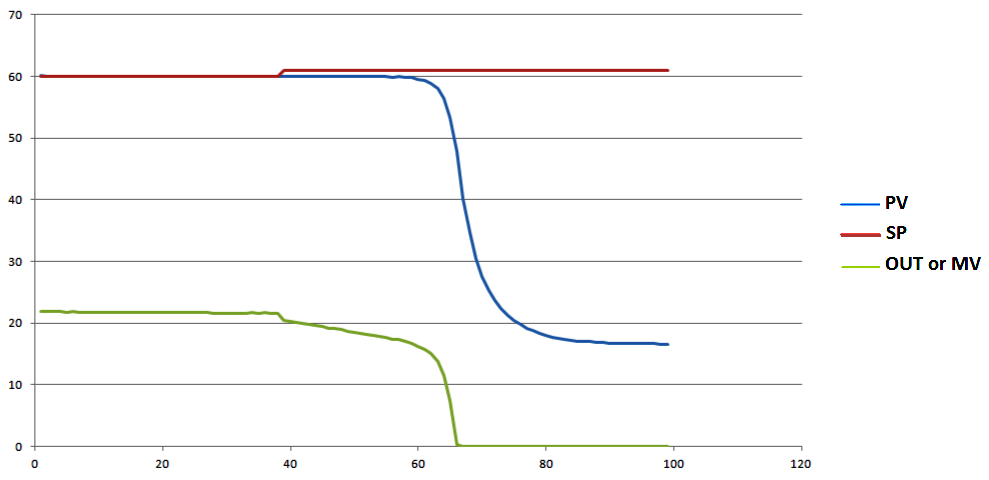
4. Examine this process trend showing the PV, SP, and Output of a loop controller:

Based on what you see here, determine the following:

• Whether this is an open-loop or a closed-loop response
• Whether the controller is (or needs to be) direct-acting or reverse-acting
• If possible, identify any problems with the field instrumentation
• If possible, identify any problems with the controller PID tuning
• Qualitatively identify the kind of PID tuning we will need for robust control

Answer :

This is a controller that has been configured for the wrong action! The configured action is direct, as evidenced both by the output’s step down as the setpoint steps up, and also the output’s downward fall as the process variable falls.

The process clearly demands a controller with reverse action in order to be regulated.

We can also see evidence of some hysteresis in the final control element. Note the small setpoint stepchange made just prior to the “40” mark on the time axis, causing the controller’s integral action to slowly wind in the downward direction.

However, the process variable does not begin to move until somewhere around the “48” time index. This suggests a final control element that is unresponsive to the change in controller output during that span of 8 time units.

The fact that the process variable plummets simultaneously with the plummeting controller output around “53” on the time axis tells us that 8-unit period of unresponsiveness is not dead time due to transport delay or compounded process lags, but that it must be due to hysteresis in the final control element.

If the 8-unit delay were due to an inherent dead-time response in the process itself, we would see the process variable’s plummeting happening 8 time units after the output signal’s downward dive.

5. Examine this process trend showing the PV, SP, and Output of a loop controller:

Based on what you see here, determine the following:

• Whether this is an open-loop or a closed-loop response
• Whether the controller is (or needs to be) direct-acting or reverse-acting
• If possible, identify any problems with the field instrumentation
• If possible, identify any problems with the controller PID tuning
• Qualitatively identify the kind of PID tuning we will need for robust control

Answer :

This is a closed-loop test, based on the fact the output signal responds dynamically to the changing process variable, as well as to the step-change in setpoint.

This is a reverse-acting controller: the output steps up when the setpoint steps up (implying the output would step down if the process variable stepped up).

There do not appear to be any field instrumentation problems revealed in this trend. A manual-mode (open-loop) test would be more informative in that regard, but it appears as though the process is very quick to respond with no discernable dead time or other lags.

The controller tuning is clearly lacking integral action. Note the large offset between PV and SP (i.e. how the process variable never settles at the setpoint value). This tells us the controller is configured only for proportional action, and this process needs integral! We can also tell this from the 180 deg phase shift between PV and output during the oscillations: this is the classic response of a reverse-acting proportional-only controller.

The actual amount of gain appears to be appropriate for the loop, since the oscillations are not excessive. We desperately need to apply some integral action to this loop, because self-regulating loops absolutely need integral action to handle load changes and achieve new setpoint values.

6. Examine this process trend showing the PV, SP, and Output of a loop controller:

Based on what you see here, determine the following:

• Whether this is an open-loop or a closed-loop response
• Whether the controller is (or needs to be) direct-acting or reverse-acting
• If possible, identify any problems with the field instrumentation
• If possible, identify any problems with the controller PID tuning
• Qualitatively identify the kind of PID tuning we will need for robust control

Answer :

This is a closed-loop test, based on the fact the output signal responds dynamically to the changing process variable, as well as to the step-change in setpoint.

This is a reverse-acting controller: the output steps up when the setpoint steps up (implying the output would step down if the process variable stepped up).

There do not appear to be any field instrumentation problems revealed in this trend. A manual-mode (open-loop) test would be more informative in that regard, but it appears as though the process is very quick to respond with no discernible dead time or other lags.

The controller tuning is too aggressive, as evidenced by the process variable’s oscillations following every setpoint change. Furthermore, we can tell this oscillation is a form of “porpoising” because it occurs prior to crossing setpoint.

Integral mode is incapable of causing a control loop to porpoise, and so the culprit must either be excessive gain (P) or excessive derivative action (D). Judging by the phase shift between process variable and setpoint (it looks to be exactly 180 degrees, with every positive PV peak matched by a negative Output peak, and vice-versa) it would appear that excessive gain (too much P action) is the cause.

This process appears to be self-regulating (as evidenced by different output values being necessary to stabilize the process variable at every new setpoint value), and there seems to be negligible time lags or dead time. This suggests a controller action that is predominantly based on integral (reset, or I) should work well, with just enough proportional action (P) to give fast response to setpoint and load changes.

Credits :  by Tony R. Kuphaldt