DC Circuit Analysis Node Equations

All of the rules governing DC circuits that have been discussed so far can now be applied to analyze complex DC circuits. To apply these rules effectively, loop equations, node equations, and equivalent resistances must be used.

Node Equations

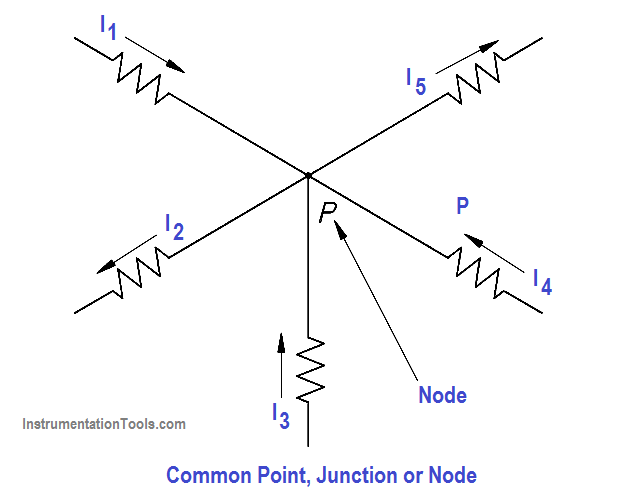
Kirchhoff’s current law, as previously stated, says that at any junction point in a circuit the current arriving is equal to the current leaving. Let us consider five currents entering and leaving a junction shown as P (Figure 43). This junction is also considered a node.

Assume that all currents entering the node are positive, and all currents that leave the node are negative. Therefore, I1 ,I3 , and I4 are positive, and I2 and I5 are negative. Kirchhoff’s Law also states that the sum of all the currents meeting at the node is zero. For Figure 43, Below Equation represents this law mathematically.

I1 + I2 + I3 + I4 + I5 = 0

Figure 43 Node Point

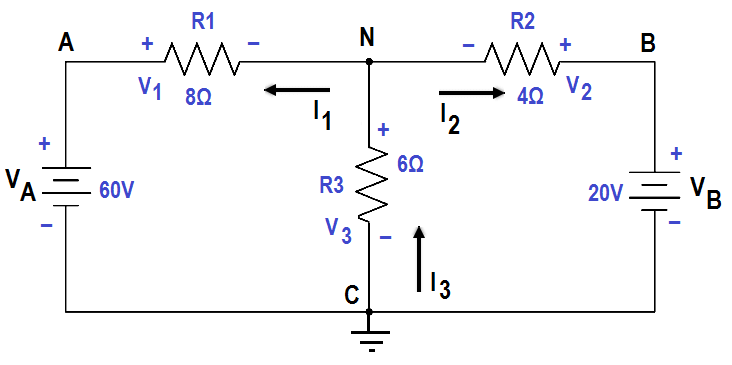
By solving node equations, we can calculate the unknown node voltages. To each node in a circuit we will assign a letter or number. In Figure 44, A, B, C, and N are nodes, and N and C are principal nodes. Principal nodes are those nodes with three or more connections. Node C will be our selected reference node.

V_AC is the voltage between Nodes A and C; V_BC is the voltage between Nodes B and C; and V_NC is the voltage between Nodes N and C. We have already determined that all node voltages have a reference node; therefore, we can substitute V_A for V_AC , V_B for V_BC , V_N for V_NC.

Figure 44 Circuit for Node Analysis

Assume that loop currentsI1 and I2 leave Node N, and that I3 enters Node N (Figure 44).

From Kirchhoff’s current law:

∑ I = 0

I1 + I2 + I3 = 0

I3 = I1 + I2

Using Ohm’s Law and solving for the current through each resistor we obtain the following.

Substitute these equations for I1 ,I2 , and I3 into Kirchhoff’s current equation yields the following.

The circuit shown in Figure 45 can be solved for voltages and currents by using the node-voltage analysis.

Figure 45 Node – Voltage Analysis

First, assume direction of current flow shown. Mark nodes A, B, C, and N, and mark the polarity across each resistor.

Second, using Kirchhoff’s current law at Node N, solve for V_N.

Clear the fraction so that we have a common denominator:

4V_N = 3 (60 – V_N) + 6 (20 – V_N)

4V_N= 180 – 3V_N + 120 – 6V_N

13V_N = 300

V_N = 23.077

Third, find all voltage drops and currents.

V₁ = V_A – V_N = 60 – 23.077 = 36.923 Volts

V₂ = V_N = 23.077 Volts

V₃ = V_B – V_N = 20 – 23.077 = -3.077 Volts

The negative value for V₃ shows that the current flow through R₃ is opposite that which was assumed and that the polarity across R₃ is reversed.

I1 = V1/R1 = 36.923 / 8 = 4.65 amp

I2 = V2/R2 = 23.077 / 6 = 3.846 amp

I3 = V3/R3 = -3.077 / 4 = – 0.769 amp

The negative value for I3 shows that the current flow through R3 is opposite that which was assumed.