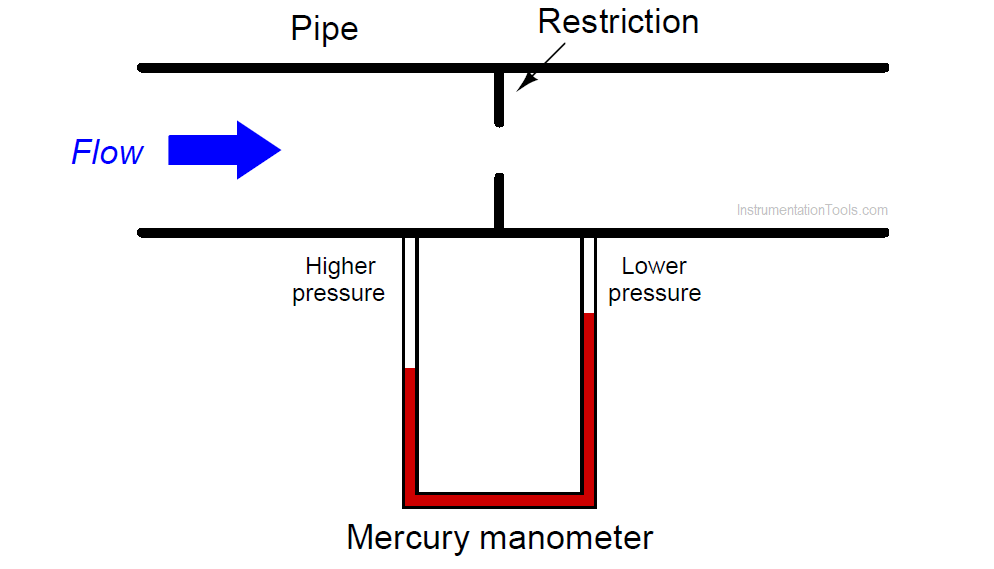
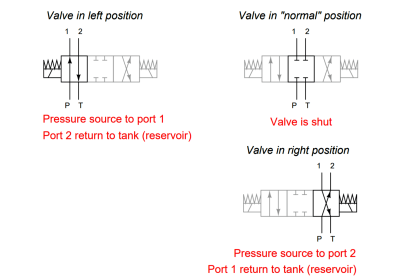
A manometer may be used to measure differential pressure across a restriction placed within a pipe. Pressure will be dropped as a result of flow through the pipe, making the manometer capable of (indirectly) measuring flow:
In the example shown above, the fluid moving through the pipe is air, and the manometer uses mercury as the indicating liquid. If we try to measure the flow rate of a liquid such as water using the same technique, though, we will find that the manometer does not register quite the way we might expect:
That is to say, given the exact same amount of differential pressure generated by the restriction, the manometer will register differently than if it was measuring air pressure.
Determine whether the manometer will register falsely high or falsely low, and also why it will do so?
Answer:
The manometer will register falsely high, showing greater differential pressure than what is actually there. If you are having difficulty figuring this out, imagine if the liquid moving through the pipe was just as dense as the mercury within the manometer: what would that do to the mercury in the manometer given any applied ∆P?
In other words, set up a thought experiment with absurdly (simple) conditions and then look for patterns or trends which you may generalize for any condition.
Challenge question: derive a mathematical correction factor for interpreting the manometer’s indication to yield true inches of mercury ∆P.
Read Next:
- What is an Orifice Flange?
- Tube and tube fittings
- Seal Material Selection
- Data Flow Communication
- Level Control Loop Problem
Credits: Tony R. Kuphaldt