Now let us consider a scenario where the pressure drop across the valve changes as the rate of flow through the valve changes.
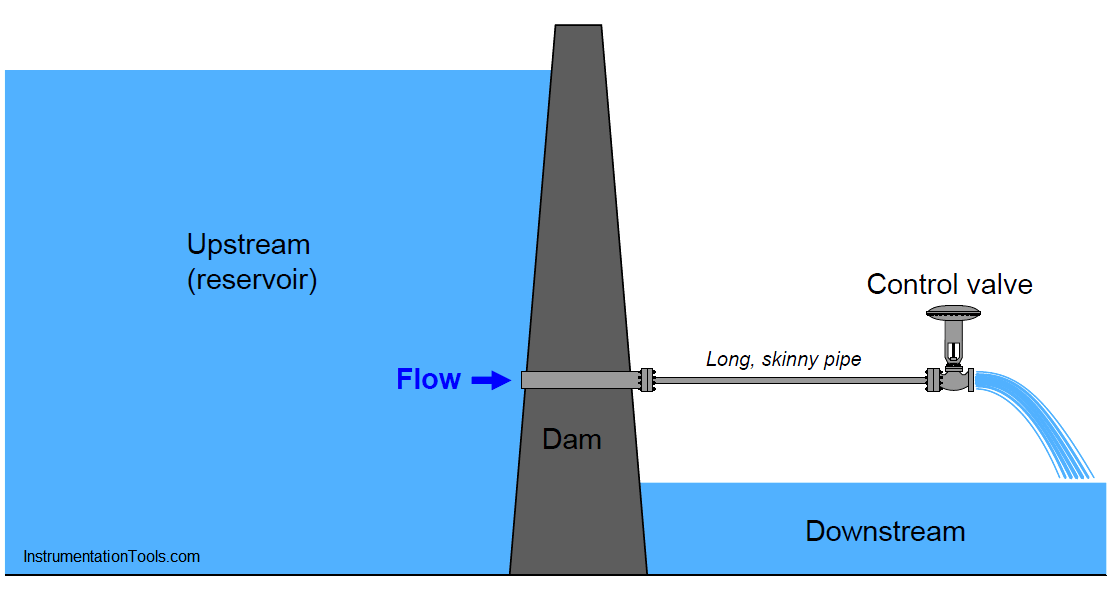
We may modify the previous example of a control valve releasing water from a dam to generate this effect. Suppose the valve is not closely coupled to the dam, but rather receives water through a narrow (restrictive) pipe:
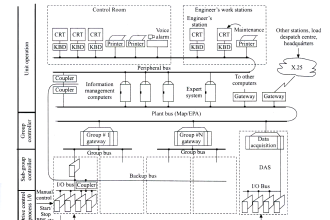
Control Valve Performance with Varying Pressure
In this installation, the narrow pipe generates a flow-dependent pressure drop due to friction between the turbulent water and the pipe walls, leaving less and less upstream pressure at the valve as flow increases.
The control valve still drains to atmosphere, so its downstream pressure is still a constant 0 PSIG, but now its upstream pressure diminishes with increasing flow. How will this affect the valve’s performance?
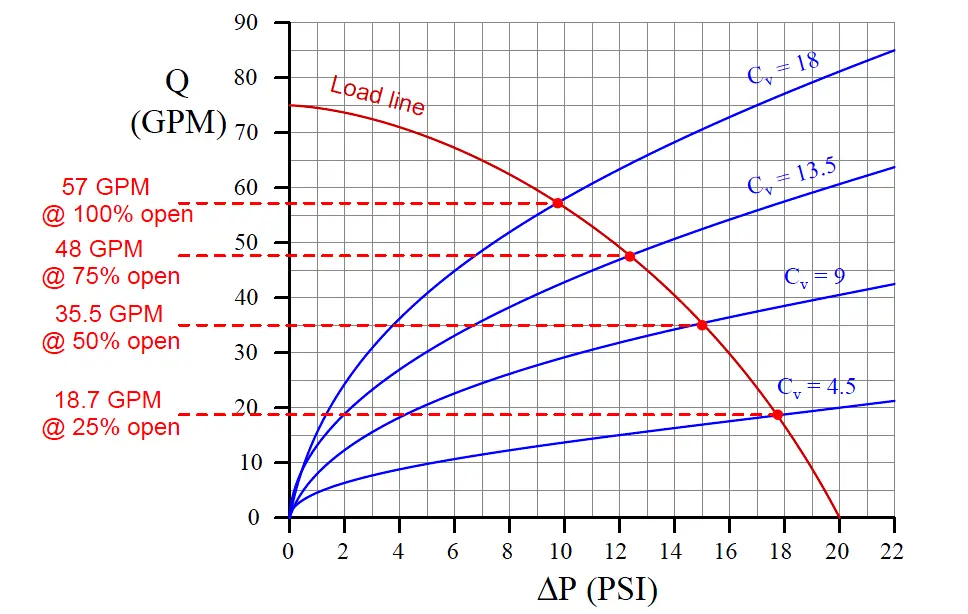
We may turn to the same set of characteristic curves to answer this question. All we need is a new load line describing the pressure available to the valve at different flow rates, then we may look for the points of intersection between this load line and the valve’s characteristic curves.
For the sake of our hypothetical example, I have sketched an arbitrary “load line” (actually a load curve) showing how the valve’s pressure falls off as flow rises (Note 1):
Note 1 : The precise determination of this curve is based on a model of the narrow pipe as a flow-restricting element, similar in behavior to an orifice, or to a control valve with a fixed stem position.
Since pressure is dropped along the pipe’s length as a function of turbulence (velocity), the load “line” curves for the exact reason the valve’s own characteristic plots are curved: the relationship between fluid velocity and turbulent pressure loss is naturally non-linear.
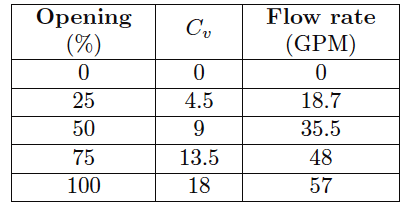
Now we see a definite non-linearity in the control valve’s behavior. No longer does a doubling of stem position (from 25% to 50%, or from 50% to 100%) result in a doubling of flow rate (Note 2):
Note 2 : Not only is the response of the valve altered by this degradation of upstream pressure, but we can also see from the load line that a certain maximum flow rate has been asserted by the narrow pipe which did not previously exist: 75 GPM.
Even if we unbolted the control valve from the pipe and let water gush freely into the atmosphere, the flow rate would saturate at only 75 GPM because that is the amount of flow where all 20 PSI of hydrostatic “head” is lost to friction in the pipe.
Contrast this against the close-coupled scenario, where the load line was vertical on the graph, implying no theoretical limit to flow at all! With an absolutely constant upstream pressure, the only limit on flow rate was the maximum Cv of the valve (analogous to a perfect electrical voltage source with zero internal resistance, capable of sourcing any amount of current to a load).
If we plot the valve’s performance in both scenarios (close-coupled to the dam, versus at the end of a restrictive pipe), we see the difference very clearly:
The “drooping” graph shows how the valve responds when it does not receive a constant pressure drop throughout the flow range. This is how the valve responds when installed in a non-ideal process, compared to the straight-line response it exhibits under ideal conditions of constant pressure. This what we mean by “installed” characteristic versus “ideal” or “inherent” characteristic.
Pressure losses due to fluid friction as it travels down pipe is just one cause of valve pressure changing with flow. Other causes exist as well, including pump curves (Note 3) and frictional losses in other system components such as filters and heat exchangers.
Whatever the cause, any piping system that fails to provide constant pressure across a control valve will “distort” the valve’s inherent characteristic in the same “drooping” manner, and this must be compensated in some way if we desire linear response from the valve.
Note 3 : The amount of fluid pressure output by any pump tends to vary with the fluid flow rate through the pump as well as the pump speed. This is especially true for centrifugal pumps, the most common pump design in process industries.
Generally speaking, the discharge (output) pressure of a pump rises as flow rate decreases, and falls as flow rate increases. Variations in system fluid pressure caused by the pump constitutes one more variable for control valves to contend with.
Not only does the diminishing pressure drop across the valve mean we cannot achieve the same full-open flow rate as in the laboratory (with a constant pressure drop), but it also means the control valve responds with different amounts of sensitivity at various points along its range.
Note how the installed characteristic graph is relatively steep at the beginning where the valve is nearly closed, and how the graph grows “flatter” at the end where the valve is nearly full-open.
The rate of response (rate-of-change of flow Q compared to stem position x, which may be expressed as the derivative dQ/dx ) is much greater at low flow rates than it is at high flow rates, all due to diminished pressure drop at higher flow rates. This means the valve will respond more “sensitively” at the low end of its travel and more “sluggishly” at the high end of its travel.
From the perspective of a feedback control system, this varying valve responsiveness means the system will be unstable at low flow rates and unresponsive at high flow rates. At low flow rates – where the valve is nearly closed – any small movement of the valve stem will have a relatively large effect on fluid flow.
However, at high flow rates, a much greater stem motion will be required to achieve a comparable effect on fluid flow. Thus, the control system will tend to over-react at low flow rates and under-react at high flow rates, simply because the control valve fails to exert the same degree of control over process flow at different flow rates. Oscillations may occur at low flow rates, and excessive deviations from setpoint at high flow rates as a result of this “distorted” valve behavior.