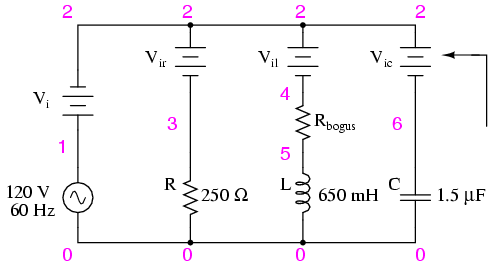
With the notable exception of calculations for power (P), all AC circuit calculations are based on the same general principles as calculations for DC circuits. The only significant difference is that fact that AC calculations use complex quantities while DC calculations use scalar quantities.
Ohm’s Law, Kirchhoff’s Laws, and even the network theorems learned in DC still hold true for AC when voltage, current, and impedance are all expressed with complex numbers. The same troubleshooting strategies applied toward DC circuits also hold for AC, although AC can certainly be more difficult to work with due to phase angles which aren’t registered by a handheld multimeter.
Power is another subject altogether, and will be covered in its own chapter in this book. Because power in a reactive circuit is both absorbed and released — not just dissipated as it is with resistors — its mathematical handling requires a more direct application of trigonometry to solve.
When faced with analyzing an AC circuit, the first step in analysis is to convert all resistor, inductor, and capacitor component values into impedances (Z), based on the frequency of the power source. After that, proceed with the same steps and strategies learned for analyzing DC circuits, using the “new” form of Ohm’s Law: E=IZ ; I=E/Z ; and Z=E/I
Remember that only the calculated figures expressed in polar form apply directly to empirical measurements of voltage and current. Rectangular notation is merely a useful tool for us to add and subtract complex quantities together. Polar notation, where the magnitude (length of vector) directly relates to the magnitude of the voltage or current measured, and the angle directly relates to the phase shift in degrees, is the most practical way to express complex quantities for circuit analysis.