Tuning a Temperature Process Control Loop
Ziegler-Nichols open-loop tuning procedure
This next simulated process is a temperature control process. Performing an open-loop test (two 10% increasing output step-changes, both made in manual mode) on this process resulted in the following behavior:
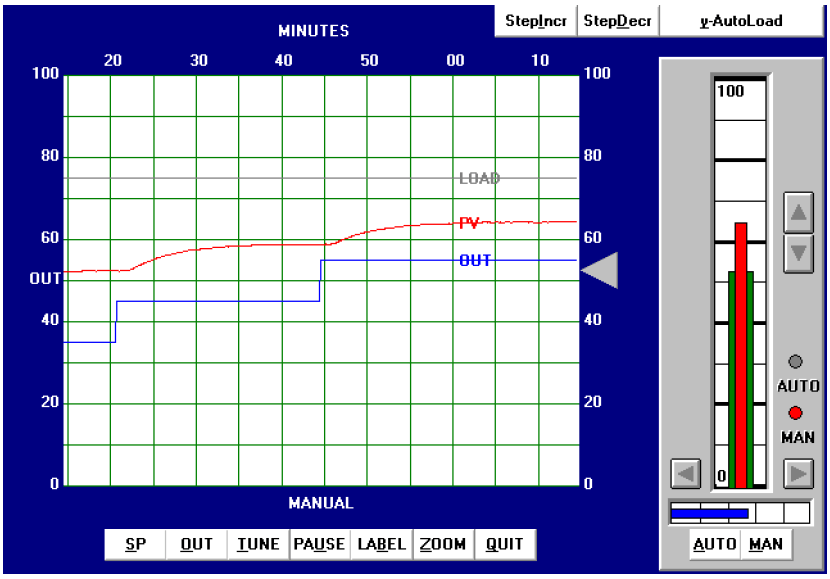
From the trend, the process appears to be self-regulating with a slow time constant (lag) and a substantial dead time. The reaction rate (R) on the first step-change is 30% over 30 minutes, or 1 percent per minute. Dead time (L) looks to be approximately 1.25 minutes. Following the Ziegler- Nichols recommendations for PID tuning based on these process characteristics (also including the 10% step-change magnitude Δm):
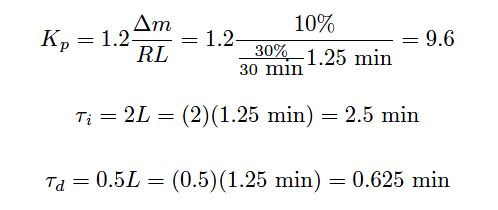
Applying the PID values of 9.6 (gain), 2.5 minutes per repeat (integral), and 0.625 minutes (derivative) gave the following result in automatic mode:
As you can see, the results are quite poor. The PV is still oscillating with a peak-to-peak amplitude of almost 20% from the last process upset at the time of the 10% downward SP change. Additionally, the output trend is rather noisy, indicating excessive amplification of process noise by the controller.
Also Read : Ziegler-Nichols Open-Loop Theory
Ziegler-Nichols closed-loop tuning procedure
Next, the closed-loop, or “Ultimate” tuning method of Ziegler and Nichols was applied to this process. Eliminating both integral and derivative control actions from the controller, and experimenting with different gain (proportional) values until self-sustaining oscillations of consistent amplitude were obtained, gave a gain value of 15:

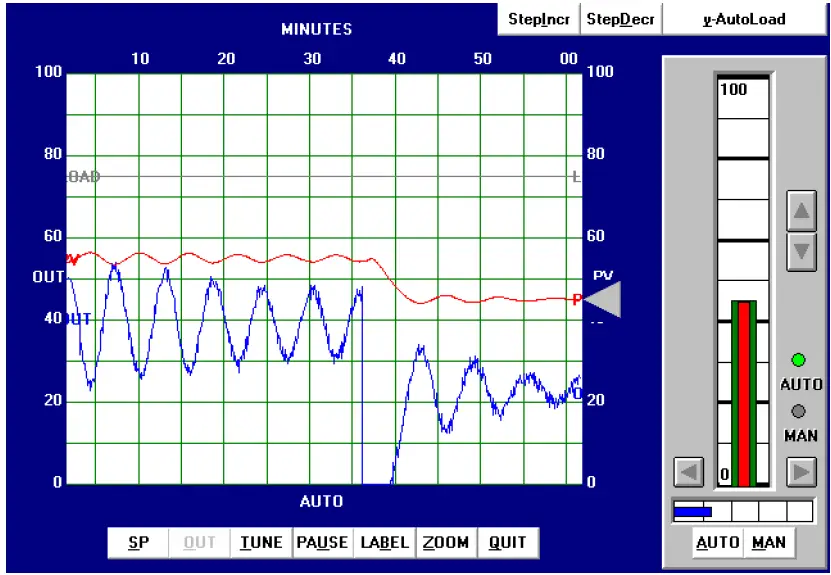
From the trend, we can see that the ultimate period (Pu) is approximately 5.2 minutes in length. Following the Ziegler-Nichols recommendations for PID tuning based on these process characteristics:

These PID tuning values are quite similar to those predicted by the open loop (“Reaction Rate”) method, and so we would expect to see very similar results:
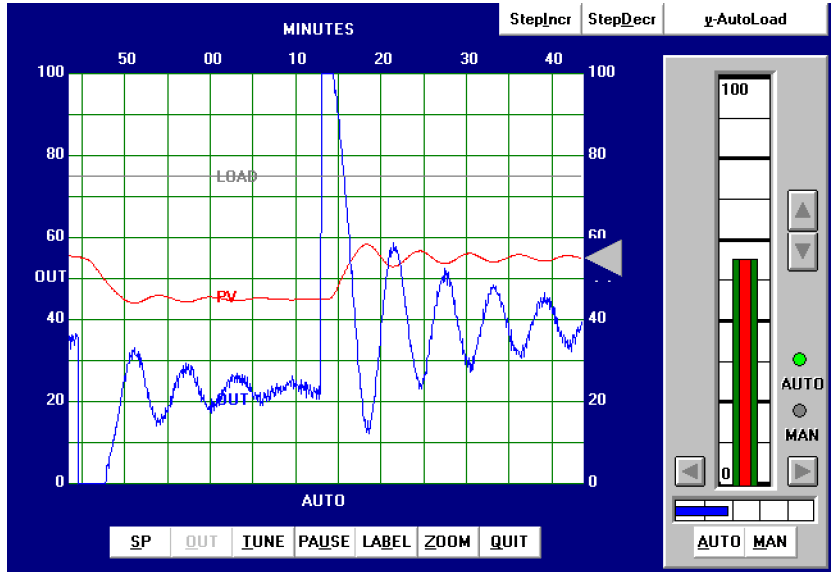
As expected, we still see excessive oscillation following a 10% setpoint change, as well as excessive “noise” in the output trend.
Also Read : Ziegler-Nichols Closed-Loop Theory
Heuristic tuning procedure
From the initial open-loop (manual output step-change) test, we could see this process was selfregulating with a slow lag and substantial dead time. The self-regulating nature of the process demands at least some integral control action to eliminate offset, but too much will cause oscillation given the long lag and dead times. The existence of over 1 minute of process dead time also prohibits the use of aggressive proportional action. Derivative action, which is generally useful in overcoming lag times, will cause problems here by amplifying process noise. In summary, then, we would expect to use mild proportional, integral, and derivative tuning values in order to achieve good control with this process. Anything too aggressive will cause problems for this process.
After some experimenting, the values I arrived at were 3 (gain), 5 minutes (integral), and 0.5 minutes (derivative). These tuning values represent a proportional action only one-third as aggressive as the Ziegler-Nichols recommendation, and an integral action about half as aggressive as the Ziegler- Nichols recommendation. The results of these tuning values in automatic mode are shown here:
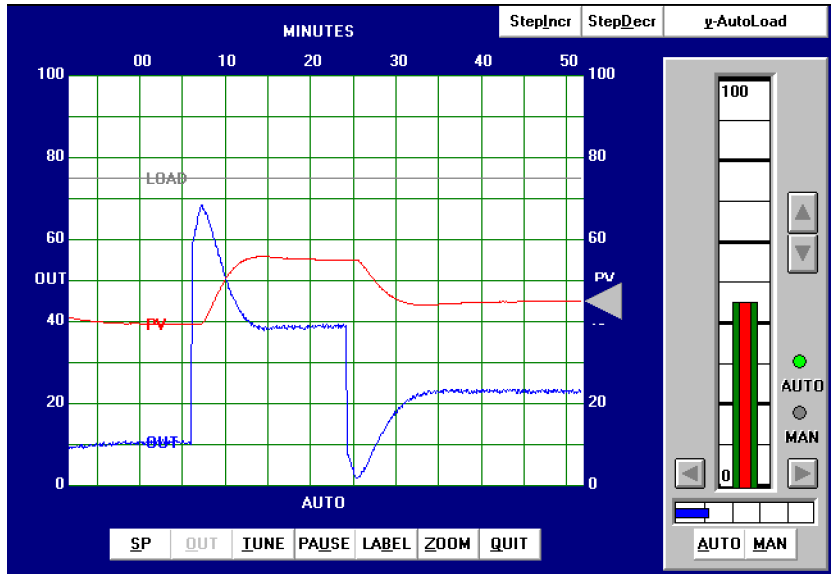
As you can see, the system’s response has almost no overshoot (with either a 10% setpoint change or a 15% setpoint change) and very little “noise” on the output trend. Response to setpoint changes is relatively crisp considering the naturally slow nature of the process: each new setpoint is achieved within about 7.5 minutes of the step-change.
Also Read : Heuristic PID Tuning Theory